Prism Definition

A prism is a three-dimensional geometric shape that is enclosed by two parallel and congruent polygonal bases and is joined by a collection of rectangular faces or sides. The base of a prism is often a polygon, and the faces or sides of the prism are formed by extending the polygonal base vertically. The prism's faces, each of which is a parallelogram, are parallel to one another and perpendicular to the bases.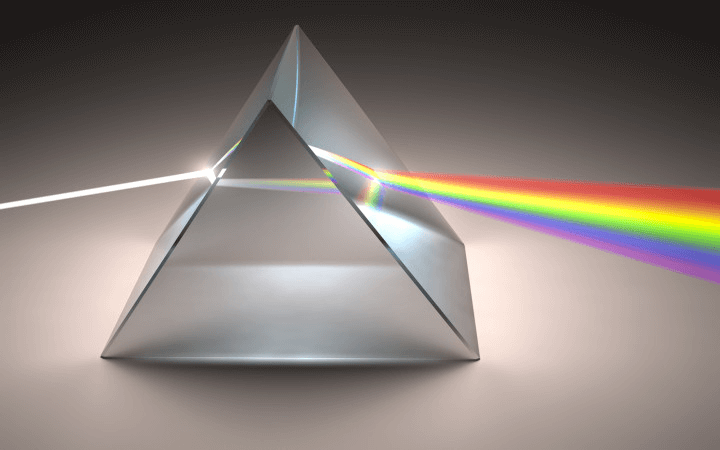

The area of the base times the height of the prism can be used to determine a prism's volume. This equation is written as V = Bh, where V is the volume of the prism, B is the area of the base, and h is the height of the prism. Prisms are used in a wide variety of real-world contexts, such as optics, architecture, and science. A prism is a transparent piece of equipment used in optics to bend or refract light. In order to divide light into its individual hues and provide a more vivid and realistic image, prisms are frequently employed in cameras and binoculars.

Working of Prism

A prism is a transparent, heavy device used to bend and scatter light. It is frequently employed in optical applications like spectroscopy, photography, and astronomy. The fundamental operation of a prism is based on the concept of refraction, which states that when light travels from one medium to another, its direction changes.

The most typical sort of prism is a triangular prism, which is a solid block of glass or plastic with two parallel and congruent triangle bases and three rectangular faces. A light beam is twisted as it enters and leaves a prism when it passes through one. As a result, the dispersion of the light's many colours occurs.

The angle of incidence, the angle between the incoming light and the prism surface, and the refractive index of the prism material-a measurement of how much the substance bends light-all affect how much refraction and dispersion occur. Snell's law, which states that the ratio of the sines of the angles of incidence and refraction equals the ratio of the refractive indices of the two media, relates the angles of incidence and refraction.

For Example- Let's Imagine a beam of white light-which consists of every color in the visible spectrum-entering one face of a triangular prism at an angle to help you comprehend how a prism works. As light enters the prism, it is bent towards the normal, a hypothetical line parallel to the prism's surface. The angle of incidence and refractive index of prism material both affect how much light is refracted.

Because each hue has a slightly distinct refractive index, the white light beam is divided into its component colors once it enters the prism. The wavelength of red light is the longest and is bent the least, whereas the wavelength of violet light is the shortest and is bent the most. Due to the various hues of the spectrum being bent at varying angles, a rainbow-like pattern of colors is produced.

Because the angle of incidence is greater than the critical angle, which is the angle at which the light is refracted at an angle of 90 degrees, the light that has been dispersed and exits the prism is refracted once more, but this time in a direction different from the normal. In order to magnify and invert the image of the item being seen via the prism, the refracted light emerges from it at an angle that is greater than the angle of incidence.

Types of Prism

Prisms are solid geometric shapes with two congruent and parallel bases that are connected by parallelogram faces. Prisms are characterized by their bases, which can be either polygons or circles. The different types of prisms and their applications are discussed below:

1. Rectangular Prism: Four rectangle-shaped faces and two rectangle-shaped bases make up a rectangular prism. As each of its lateral sides is perpendicular to each of its bases, it is a right prism. To reflect and invert pictures in binoculars and periscopes, a rectangular prism is frequently employed in optics. Moreover, walls and floors can be constructed using it.
2. Triangular Prism: Two triangle-shaped bases and three rectangle-shaped faces make up a triangle-shaped prism. All of its lateral faces are parallel to its bases, making it a right prism as well. A triangular prism is frequently used in optics to refract light and divide white light into its component colours. Moreover, as a structural component, it is utilised in architecture and construction.
3. Pentagonal Prism: A pentagonal prism has five rectangular faces and two pentagonal bases. Due to the fact that each of its lateral faces is perpendicular to each of its bases, it is also a right prism. In optics, a pentagonal prism is frequently used to bend light and produce rainbow effects. Moreover, it serves as a decorative component in architecture and construction.
4. Hexagonal Prism: Two hexagonal bases and six rectangular faces make up a hexagonal prism. Due to the fact that each of its lateral faces is perpendicular to each of its bases, it is also a right prism. In optics, a hexagonal prism is frequently used to refract light and produce prism effects. It serves as a structural component in both architecture and construction.
5. Trapezoidal Prism: A trapezoidal prism has two trapezoidal bases and four rectangular faces. It is not a right prism because its lateral faces are not perpendicular to its bases. A trapezoidal prism is commonly used in optics to refract light and separate white light into its constituent colors. It is also used in architecture and construction as a structural element.
6. Rhomboidal Prism: A rhomboidal prism has two rhomboidal bases and four rhomboidal faces. It is not a right prism because its lateral faces are not perpendicular to its bases. A rhomboidal prism is commonly used in optics to refract light and create prism effects. It is also used in architecture and construction as a decorative element.
7. Octagonal Prism: An octagonal prism has two octagonal bases and eight rectangular faces. It is also a right prism because all its lateral faces are perpendicular to its bases. An octagonal prism is commonly used in optics to refract light and create prism effects. It is also used in architecture and construction as a structural element.

Uses of Prism

Prisms are used to refract and reflect light, separate white light into its constituent colors, invert images, create optical illusions, and more.

1. Optics: Prisms are extensively used in optics for a wide range of applications, such as binoculars, cameras, microscopes, telescopes, and laser devices. One of the primary uses of prisms in optics is to refract light and bend it at specific angles. For instance, a prism can be used to split a beam of white light into its component colors, creating a spectrum.

Prisms are also used to correct chromatic aberrations in lenses by refracting the light in such a way that all colors converge at the same point, resulting in sharper images. Another common use of prisms in optics is to invert and reflect images, as in the case of periscopes and binoculars.

2. Spectroscopy: Spectroscopy is a scientific technique used to study the properties of light and matter. Prisms are commonly used in spectroscopy to disperse light into its constituent colors, creating a spectrum that can be analyzed to identify the chemical composition of a material.

The spectrum produced by a prism can reveal information about the atoms and molecules present in a sample, including their energy levels, electronic structure, and bonding properties. Prisms are also used in other spectroscopic techniques, such as infrared spectroscopy and X-ray diffraction, to analyze the properties of light in different regions of the electromagnetic spectrum.

3. Photography: Prisms are used in photography to create various optical effects and enhance the quality of images. One of the most common uses of prisms in photography is to create prism effects, which involve refracting light in such a way that it creates colorful patterns and shapes.

Prisms can also be used to create kaleidoscope effects, where multiple images are reflected and merged to create a symmetrical pattern. Another use of prisms in photography is to invert and reflect images, as in the case of periscopes and binoculars.

4. Physics: Prisms are used in physics to study the properties of light, such as its speed, wavelength, and polarization. Prisms can be used to split a beam of light into two or more beams, each with different properties, such as different wavelengths or polarizations. By analyzing the properties of these beams, physicists can gain insights into the fundamental nature of light and its interactions with matter.

5. Medicine: Prisms are used in medicine to diagnose and treat various vision disorders, such as strabismus and diplopia. Strabismus is a condition in which the eyes are misaligned, causing double vision. Prisms can be used to correct this condition by refracting light in such a way that it brings the images from the two eyes into alignment.

Diplopia, or double vision, is another vision disorder that can be corrected using prisms. Prisms can be used to shift the position of the images in the two eyes, reducing or eliminating the double vision.

6. Architecture: Prisms are used in architecture to create various decorative and functional elements. For instance, prisms can be used to create a stained glass effect by refracting and diffusing light in colorful patterns.

Prisms can also be used to create skylights and light wells that allow natural light to penetrate deep into buildings. In addition, prisms can be used as structural elements to support roofs and walls, as in the case of vaulted ceilings and domes.

Important Formulas

A prism is a solid figure with two parallel and congruent bases that are connected by a set of rectangular faces. The volume of a prism is calculated by multiplying the area of the base by the height of the prism. The surface area of a prism is calculated by adding up the area of each face of the prism.

Calculating the Volume of a Prism:

To calculate the volume of a prism, you need to know the area of the base and the height of the prism. The formula for finding the volume of a prism is:

Volume of Prism = Area of Base x Height

For example, if the base of the prism is a rectangle with a length of 4 cm and a width of 6 cm, and the height of the prism is 8 cm, then the volume of the prism would be:

The volume of Prism = 4 cm x 6 cm x 8 cm = 192 cm³

Calculating the Surface Area of a Prism:

To calculate the surface area of a prism, you need to know the area of each face of the prism. The formula for finding the surface area of a prism is:

Surface Area of Prism = 2 x Area of Base + Perimeter of Base x Height

For example, if the base of the prism is a rectangle with a length of 4 cm and a width of 6 cm, and the height of the prism is 8 cm, then the surface area of the prism would be: Surface Area of Prism = 2 x (4 cm x 6 cm) + (4 cm + 6 cm) x 8 cm = 96 cm² + 80 cm² = 176 cm²

Calculating the Area of the Base of a Prism:

The area of the base of a prism can be calculated using the formula for the area of the shape that makes up the base of the prism. For example, if the base of the prism is a rectangle with a length of 4 cm and a width of 6 cm, then the area of the base of the prism would be:

Area of Base = 4 cm x 6 cm = 24 cm²

The calculation of prisms is an important aspect of geometry. The volume and surface area of a prism can be calculated using simple formulas based on the shape of its base and height. Prisms have various applications in different fields and are essential in the development of technologies and designs.

Concept of Refractive Index by Prism

The refractive index of a medium is a measure of how much light is bent when it passes through that medium. When light passes through a prism, it is refracted, or bent, at an angle that depends on the refractive index of the prism. The calculation of the refractive index of a prism involves measuring the angles of incidence and refraction of a beam of light passing through the prism and using Snell's law.

Snell's Law: Snell's law is a formula that relates the angle of incidence of a beam of light to the angle of refraction as it passes through a boundary between two media with different refractive indices. The formula is:

n1 sinθ1 = n2 sinθ2

where n1 and n2 are the refractive indices of the two media, θ1 is the angle of incidence, and θ2 is the angle of refraction.

Calculation of Refractive Index:

To calculate the refractive index of a prism, you need to measure the angles of incidence and refraction of a beam of light passing through the prism. The formula for calculating the refractive index of a prism is:

Refractive Index of Prism = (sin((A+D)/2))/(sin(A/2))

where A is the angle of the prism and D is the angle of deviation.

The angle of deviation is the angle between the incident beam and the emergent beam after passing through the prism. To measure the angle of deviation, you can use a spectrometer, which is an instrument used to measure the angles of light.