Aptitude Logarithm Test Paper 311) The value of Log10 2 + 16 log10
Answer: C Explanation: We know that log mn = n log m So, Log10 2 + log10 [24/ 3*5] 16 + log10 [52/ 23 * 3] 12 + log10 [34/ 24 * 5] 7 Or, log10 2 + log10 [264/ 316 * 516] + log10 [536/ 236 * 312] + log10 [328/ 228 * 57] We know that log m*n = log m + log n Now, log10 [2 * 264 * 524 * 328]/ [316 * 516 * 236 * 312 * 228 * 57] Or, log10 [265 * 328 *524]/ [264 * 328 *523] Therefore, log10 (2*5) = log10 (10) = 1 12) If
Answer: A Explanation: We have Now, we can write it as We know that (a+b) 2 = a2 + b2 + 2ab Similarly, Or, Both side Log will be canceled out Now, 2 + √7 = 2 + x Therefore, x = 2 + √7 - 2 = √7 13) If log10
Answer: A Explanation: We have log10 Or, we can write it as log10 Or, log10 Therefore, both side log will be canceled out.
Or, We know that √x = a, or x1/2 = a, then x = a2 Similarly, x2 - 12x + 36 = 42 Or, x2 - 12x + 36 = 16 Or, x2 - 12x + 20=0 Now, (x-2)(x-10) = 0 Or, x-2 = 0, and x-10 =0 Therefore, we can say that x = 2, x = 10 14) If f(a) = log
Answer: C Explanation: We can find it by replacing a with Now, f Or, log Now, the denominator of both fractions will cancel out each other. Or, log We know that log mn = n log m. 15) The value of log10
Answer: D Explanation: Then the output will be the number itself, i.e., the expression Since, logm m = 1 Therefore, log10 (10) = 1 Aptitude Logarithm Test Paper 1 Aptitude Logarithm Test Paper 2 Aptitude Logarithm Test Paper 4
Next TopicAptitude Logarithm Test Paper 4
|
 For Videos Join Our Youtube Channel: Join Now
For Videos Join Our Youtube Channel: Join Now
Feedback
- Send your Feedback to [email protected]
Help Others, Please Share





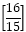 + 12 log10
+ 12 log10 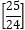 + 7 log10
+ 7 log10  is
is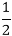 log (11 + 4√7) = log (2 + x), what is the value of x?
log (11 + 4√7) = log (2 + x), what is the value of x? 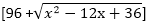 = 2, find the value of x.
= 2, find the value of x. 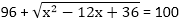
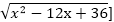 = 4
= 4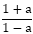 , f (2a / 1+a2) is equals to
, f (2a / 1+a2) is equals to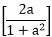
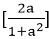 = log
= log 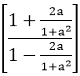
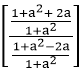
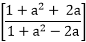
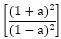 = log
= log 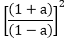
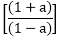 is equals to 2 f(a).
is equals to 2 f(a).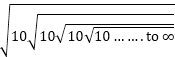 is
is




