NCERT Class 10 Maths

Chapter 4: Quadratic Equations

Exercise 4.1

1. Check whether the following are quadratic equations:

1. (x + 1)² = 2(x - 3)
2. x² - 2x = (-2) (3 - x)
3. (x - 2)(x + 1) = (x - 1)(x + 3)
4. (x - 3)(2x +1) = x(x + 5)
5. (2x - 1)(x - 3) = (x + 5)(x - 1)
6. x² + 3x + 1 = (x - 2)²
7. (x + 2)³ = 2x (x² - 1)
8. x³ - 4x² - x + 1 = (x - 2)³

Solution

I.

(x + 1)² = 2(x - 3)
x² + 1 + 2x = 2x - 6
x² + 7 = 0

Since, the highest power of x is 2. Therefore, the given equation is quadratic.

II.

x² - 2x = -2(3 - x)
x² - 2x = 2x - 6
x² - 4x + 6 = 0

Since, the highest power of x is 2. Therefore, the given equation is quadratic.

III.

(x - 2)(x + 1) = (x - 1)(x + 3)
x² + x - 2x - 2 = x² - x + 3x - 3
x² - x - 2 = x² + 2x - 3
0 = 3x - 1

Since, the highest power of x is 1. Therefore, the given equation is not quadratic.

IV.

(x - 3)(2x + 1) = x(x + 5)
2x² + x - 6x - 3 = x² + 5x
x² - 5x - 3 = 5x
x² - 10x - 3 = 0

Since, the highest power of x is 2. Therefore, the given equation is quadratic.

V.

(2x - 1)(x - 3) = (x - 1)(x + 5)
2x² - 6x - x + 3 = x² + 5x - x - 5
x² - 7x + 3 = 4x - 5
x² - 11x + 8 = 0

Since, the highest power of x is 2. Therefore, the given equation is quadratic.

VI.

x² + 3x + 1 = (x - 2)²
x² + 3x + 1 = x² - 4x + 4
7x - 3 = 0

Since, the highest power of x is 1. Therefore, the given equation is not quadratic.

VII.

(x + 2)^3 = 2x(x² - 1)
x^3 + 8 + 6x² + 12x = 2x^3 - 2x
0 = x^3 - 6x² - 14x - 8

Since, the highest power of x is 3. Therefore, the given equation is not quadratic.

VIII.

x^3 - 4x^(2 )- x + 1 = (x - 2)^3
x^3 - 4x² - x + 1 = x^3 - 6x² + 12x - 8
2x² - 13x + 9 = 0

Since, the highest power of x is 2. Therefore, the given equation is quadratic.

2. Represent the following situations in the form of quadratic equations :

1. The area of a rectangular plot is 528 m² . The length of the plot (in metres) is one more than twice its breadth. We need to find the length and breadth of the plot.
2. The product of two consecutive positive integers is 306. We need to find the integers.
3. Rohan's mother is 26 years older than him. The product of their ages (in years) 3 years from now will be 360. We would like to find Rohan's present age.
4. A train travels a distance of 480 km at a uniform speed. If the speed had been 8 km/h less, then it would have taken 3 hours more to cover the same distance. We need to find the speed of the train.

Solution

I. Let the breadth of the rectangular plot be x (in metres).

It is known that the length of the plot is one more than two times the breadth,

Therefore the length of the rectangular plot = 2x + 1

We know that,

Area of Rectangle = Length × Breadth

Hence, the required equation will be:

528 = x(2x + 1)
528 = 2x² + x
2x² + x - 528 = 0

II. Let the smaller integer be x.

It is known that both the integers are consecutive,

Therefore the other integer will be = x + 1.

It is given that the product of these two integers is 306.

Hence, the required equation will be:

306 = x(x + 1)
306 = x² + x
x² + x - 306 = 0

III. Let the present age of Rohan (in years) be x.

Then, the present age of his mother (in years) = x + 26.

It is given that the product of their ages 3 years into the future would be 360.

Hence, the required equation will be:
(x + 3)(x + 26 + 3) = 360
x² + 26x + 3x + 3x + 78 + 9 = 360
x² + 32x + 87 = 360
x² + 32x - 273 = 0

IV. Let the speed of the train (in km/h) be x.

We know that,

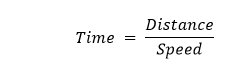
Using this knowledge, we can conclude that the time taken by the train = 480/x

It is given that reducing the speed of the train by 8 km/h would have resulted in 3 hours of extra time required for the journey.

Hence, the required equation will be:

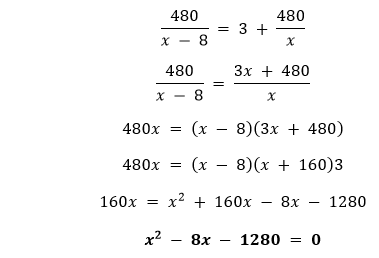
Exercise 4.2

1. Find the roots of the following quadratic equations by factorisation:

1. x² - 3x - 10 = 0
2. 2x² + x - 6 = 0
3. √2x² + 7x + 5√2 = 0
4. 2x² - x + 1/8 = 0
5. 100x² - 20x + 1 = 0

Solution

I.

x² - 3x - 10 = 0
x² - 5x + 2x - 10 = 0
x(x - 5) + 2(x - 5) = 0
(x - 5)(x + 2) = 0
x - 5 = 0
⇒ x = 5

OR

x + 2 = 0
⇒ x = -2

Hence, the roots of the given equation are 5 and -2.

II.

2x² + x - 6 = 0
2x² + 4x - 3x - 6 = 0
2x(x + 2) - 3(x + 2) = 0
(x + 2)(2x - 3) = 0
2x - 3 = 0
2x = 3
⇒ x = 3/2

OR

x + 2 = 0
⇒ x = -2

Hence, the roots of the given equation are 3/2 and -2.

III.

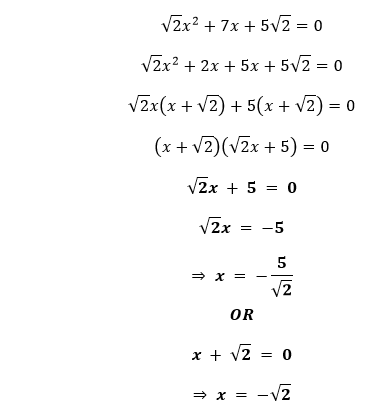
Hence, the roots of the given equation are -5/√2 and -√2.

IV.

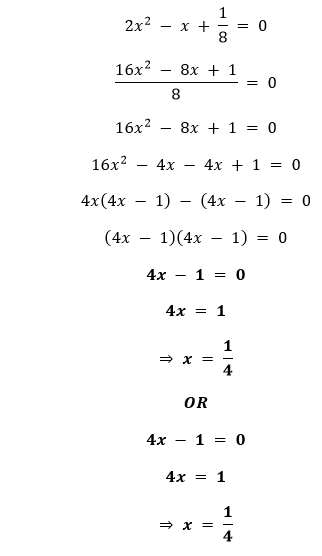
Hence, the roots of the given equations are ¼ and ¼.

V.

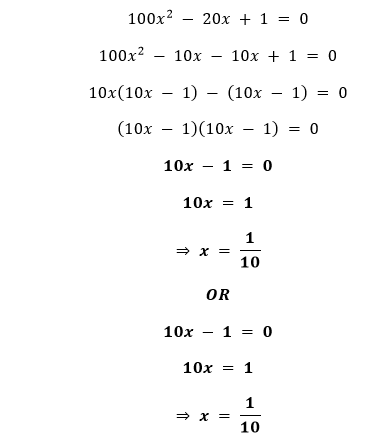
Hence, the roots of the given equations are 1/10 and 1/10.

2. Solve the problems given in Example 1.

(For reference) Problems from Example 1:

1. John and Jivanti together have 45 marbles. Both of them lost 5 marbles each, and the product of the number of marbles they now have is 124. We would like to find out how many marbles they had to start with.
2. A cottage industry produces a certain number of toys in a day. The cost of production of each toy (in rupees) was found to be 55 minus the number of toys produced in a day. On a particular day, the total cost of production was Rs 750. We would like to find out the number of toys produced on that day.

Solution

I. Let the number of marbles John had at the start be x.

Then the number of marbles Jivanti had at the start will be 45 - x.

It is given that both of them lost 4 marbles and the product of their marbles becomes 124. Therefore

(x - 5)(45 - x - 5) = 124
-(x - 4)(x - 40) = 124
x² - 40x - 5x + 160 = -124
x² - 45x + 324 = 0
x² - 9x - 36x + 324 = 0
x(x - 9) - 36(x - 9) = 0
(x - 9)(x - 36) = 0
x - 9 = 0
⇒ x = 9
45 - x = 45 - 9 = 36
OR
x - 36 = 0
⇒ x = 36
45 - x = 45 - 36 = 9

Hence, John and Jivanti had 9 and 36 marbles respectively or 36 and 9 marbles respectively.

II. Let the number of toys produced in a day by the cottage industry be x.

Then, the cost of production of each toy (in rupees) = 55 - x

It is given that on a particular day, the total cost of production was Rs 750. Therefore

Hence, the number of toys produced in the cottage industry on the given day was either 25 or 30.

3. Find two numbers whose sum is 27 and product is 182.

Solution

Let one of the two numbers be x.

Then, the other number will be = 27 - x

It is given that the product of these numbers is 182. Therefore,

x(27 - x) = 182
27x - x² = 182
x² - 27x + 182 = 0
x² - 14x - 13x + 182 = 0
x(x - 14) - 13(x - 14 )= 0
(x - 14)(x - 13) = 0
x - 14 = 0
⇒ x = 14
27 - x = 27 - 14 = 13
OR
x - 13 = 0
⇒ x = 13
27 - x = 27 - 13 = 14

Hence, the two numbers are 13 and 14 respectively or 14 and 13 respectively.

4. Find two consecutive positive integers, sum of whose squares is 365.

Solution

Let the smaller positive integer be x.

Then the other positive integer = x + 1

It is given that the sum of the squares of the given two numbers is 365. Therefore,

(x² ) + (x + 1)² = 365
x² + x² + 2x + 1 = 365
2x² + 2x - 364 = 0
2(x² + x - 182) = 0
x² + 14x - 13x - 182 = 0
x(x + 14) - 13(x + 14) = 0
(x + 14)(x - 13) = 0
x + 14 = 0
⇒ x = -14
OR
x - 13 = 0
⇒ x = 13

But, it is given to us that the integers are positive. Therefore, x = -14 is rejected.

Hence, the required positive integers are 13 and 13 + 1 = 14.

5. The altitude of a right triangle is 7 cm less than its base. If the hypotenuse is 13 cm, find the other two sides.

Solution

Let the base of the right triangle (in cm) be x.

Then, the altitude of the right triangle (in cm) = x - 7

Hypotenuse = 13 cm (Given)

According to the Pythagoras Theorem,

Base^(2 )+ Altitude² = Hypotenuse²

Therefore,

x² + (x - 7)² = 13²
x² + x² + 49 - 14x = 169
2x² - 14x - 120 = 0
2(x² - 7x - 60) = 0
x² - 12x + 5x - 60 = 0
x(x - 12) + 5(x - 12) = 0
(x - 12)(x + 5) = 0
x + 5 = 0
⇒ x = -5
OR
x - 12 = 0
⇒ x = 12

But, x is length and can never be negative. Therefore, x = -15 is rejected.

Hence, the base of the given right triangle is 12 cm and the altitude is 12 - 7 = 5 cm.

6. A cottage industry produces a certain number of pottery articles in a day. It was observed on a particular day that the cost of production of each article (in rupees) was 3 more than twice the number of articles produced on that day. If the total cost of production on that day was Rs 90, find the number of articles produced and the cost of each article.

Solution

Let the number of pottery articles produced in a day by the cottage industry be x.

Then, the cost of production of each pottery article (in rupees) = 2x + 3

It is given that on a particular day, the total cost of production was Rs 90. Therefore

x(2x + 3) = 90

(Number of articles produced in the day × Cost of each article = Total cost)

2x² + 3x = 90
2x² + 3x - 90 = 0
2x² + 15x - 12x - 90 = 0
x(2x + 15) - 6(2x + 15) = 0
(2x + 15)(x - 6) = 0
x - 6 = 0
⇒ x = 6
OR
2x + 15 = 0
2x = 15

But, the number of articles produced cannot be in fractions. Therefore, x = 15/2 is rejected.

Hence, the number of articles produced in the cottage industry on the given day was 6 and the cost of each article = 90/6 = Rs 15.

Exercise 4.3

1. Find the roots of the following quadratic equations, if they exist, by the method of completing the square:

1. 2x² - 7x + 3 = 0
2. 2x² + x - 4 = 0
3. 4x² + 4√3x + 3 = 0
4. 2x² + x + 4 = 0

Solution

I.

2x² - 7x + 3 = 0

We need the coefficient of x2 to be 1. So, we will divide both sides by 2.

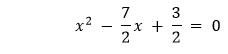
Add and subtract (7/4)² on the LHS.

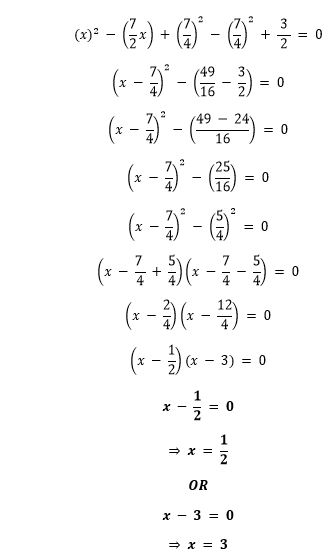
Hence, the roots are x = ½ and x = 3.

II.

2x² + x - 4 = 0

We need the coefficient of x2 to be 1. So, we will divide both sides by 2.

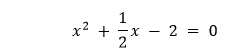
Add and subtract (1/4)² on the LHS.

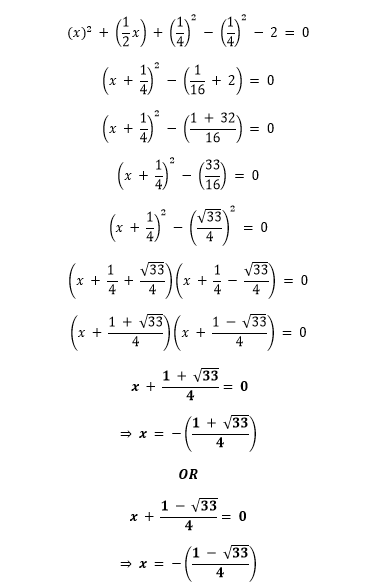
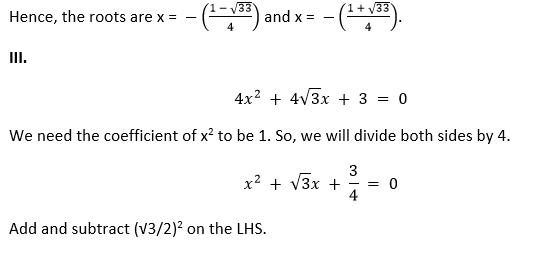
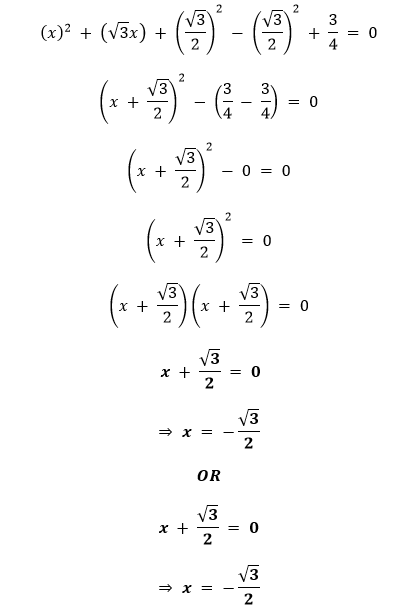
Hence, the roots are x = -√3/2 and x = -√3/2.

IV.

2x² + x + 4 = 0

We need the coefficient of x2 to be 1. So, we will divide both sides by 2.

Add and subtract (1/4)2 on the LHS.

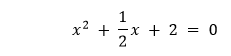
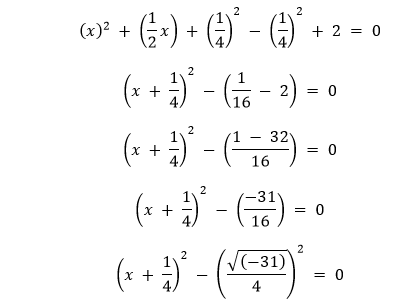
√(-31) is an imaginary number.

Hence, roots are not real for the given quadratic equation.

2. nd the roots of the quadratic equations given in Q.1 above by applying the quadratic formula.

(For reference) Equations from question 1 :

1. 2x² - 7x + 3 = 0
2. 2x² + x - 4 = 0
3. 4x² + 4 √3x + 3 = 0
4. 2x² + x + 4 = 0

Solution

I. On comparing the given quadratic equation with ax² + bx + c = 0, we get

a = 2, b = -7 and c = 3

Find the discriminant

d = b² - 4ac
d = (-7)² - 4(2)(3)
d = 49 - 24
d = 25

Since, d > 0.

Therefore, two real roots α and β exist for the given equation.

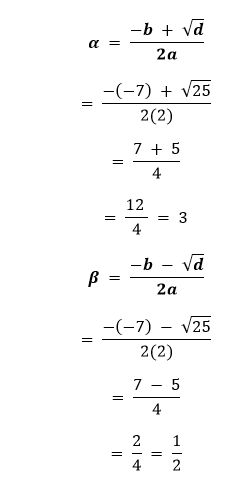
Hence, the roots are 3 and ½.

I. On comparing the given quadratic equation with ax² + bx + c = 0, we get

a = 2, b = 1 and c = -4

Find the discriminant

d = b² - 4ac
d = (1)² - 4(2)(-4)
d = 1 + 32
d = 33

Since, d > 0.

Therefore, two real roots α and β exist for the given equation.

III. On comparing the given quadratic equation with ax² + bx + c = 0, we get

a = 4, b = 4√3 and c = 3

Find the discriminant

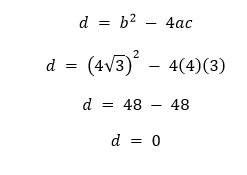
Since, d = 0.

Therefore, two equal and real roots α and β exist for the given equation.

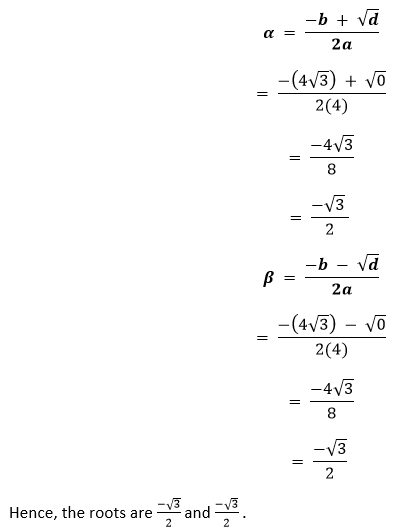
IV. On comparing the given quadratic equation with ax2 + bx + c = 0, we get

a = 2, b = 1 and c = 4

Find the discriminant

d = b² - 4ac
d = (1)² - 4(2)(4)
d = 1 - 32
d = -31

Since, d < 0.

Therefore, real roots do not exist for this equation.

3. Find the roots of the following equations:

1. x - 1/x = 3, x ≠ 0
2. 1/(x + 4) - 1/(x - 7) = 11/30, x ≠ -4, 7

Solution

I.

Multiply both sides by x.

x² - 1 = 3x

x² - 3x - 1 = 0

On comparing the given quadratic equation with ax2 + bx + c = 0, we get

a = 1, b = -3and c = -1

Find the discriminant

d = b² - 4ac
d = (-3)² - 4(1)(-1)
d = 9 + 4
d = 13

Since, d > 0.

Therefore, two real roots α and β exist for the given equation.

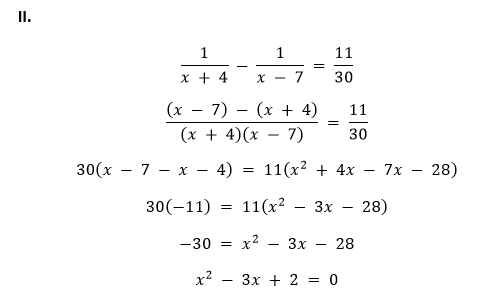
On comparing the given quadratic equation with ax2 + bx + c = 0, we get

a = 1, b = -3and c = 2

Find the discriminant

d = b² - 4ac
d = (-3)² - 4(1)(2)
d = 9 - 8
d = 1

Since, d > 0.

Therefore, two real roots α and β exist for the given equation.

Hence, the roots are 2 and 1.

4. The sum of the reciprocals of Rehman's ages, (in years) 3 years ago and 5 years from now is 1/3. Find his present age.

Solution

Let the present age of Rehman (in years) be x.

Reciprocal of his age 3 years ago = 1/(x - 3)

Reciprocal of his age 5 years later = 1/(x + 5)

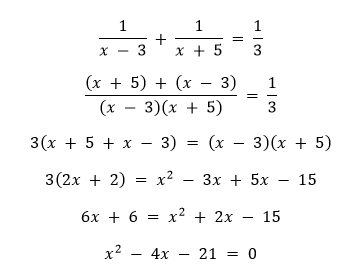
On comparing the given quadratic equation with ax² + bx + c = 0, we get

a = 1, b = -4 and c = 21

Find the discriminant

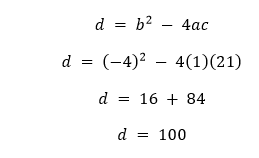
Since, d > 0.

Therefore, two real roots α and β exist for the given equation.

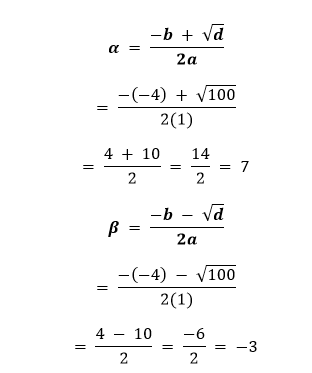
Since, age cannot be negative, therefore x = -3 is rejected.

Hence, Rehman's present age (in years) is x = 7.

5. In a class test, the sum of Shefali's marks in Mathematics and English is 30. Had she got 2 marks more in Mathematics and 3 marks less in English, the product of their marks would have been 210. Find her marks in the two subjects.

Solution

Let Shefali's marks in Mathematics be x.

Then, Shefali's marks in English = 30 - x.

It is given that if she had gotten 2 marks more in Mathematics and 3 less marks in English, the product of the marks would be 210. Therefore

(x + 2)(30 - x - 3) = 210
(x + 2)(27 - x) = 210
27x - x² + 54 - 2x = 210
-x² + 25x + 54 - 210 = 0
x² - 25x + 156 = 0

On comparing the given quadratic equation with ax2 + bx + c = 0, we get

a = 1, b = -25 and c = 156

Find the discriminant

d = b² - 4ac
d = (-25)² - 4(1)(156)
d = 625 - 624
= 1

Since, d > 0.

Therefore, two real roots α and β exist for the given equation.

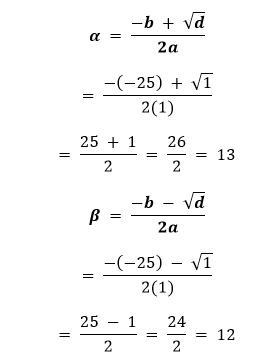
When x = 12,

30 - x = 30 - 12 = 18

When x = 13,

30 - x = 30 - 13 = 17

Hence, Shefali got either 13 marks in Mathematics and 17 marks in English OR 12 marks in Mathematics and 18 marks in English.

6. The diagonal of a rectangular field is 60 metres more than the shorter side. If the longer side is 30 metres more than the shorter side, find the sides of the field.

Solution

Let the longer side of the rectangular field (in metres) be x.

Then the shorter side of the rectangular field (in metres) = x - 30

The diagonal of the rectangle = 60 + (x - 30)

The diagonal of the rectangular field and two sides form a right triangle.

According to Pythagoras Theorem,

Hypotenuse² = Base² + Height²
(60 + x - 30)² = x² + (x - 30)²
(x + 30)² = x² + (x - 30)²
x² + 900 + 60x = x^(2 )+ (x² + 900 - 60x)
120x = x²
120 = x

Shorter side = x - 30 = 120 - 30 = 90 m

Hence, the longer side of the rectangular field is 120 m while the shorter side is 90 m.

7. The difference of squares of two numbers is 180. The square of the smaller number is 8 times the larger number. Find the two numbers.

Solution

Let the larger number = x.

Then the square of smaller number = 8x

Since, the difference of the squares of the two numbers is 180. Therefore

x² - 8x = 180
x² - 8x - 180 = 0

On comparing the given quadratic equation with ax2 + bx + c = 0, we get

a = 1, b = -8 and c = -180

Find the discriminant

d = b² - 4ac
d = (-8)² - 4(1)(-180)
d = 64 + 720
d = 784

Since, d > 0.

Therefore, two real roots α and β exist for the given equation.

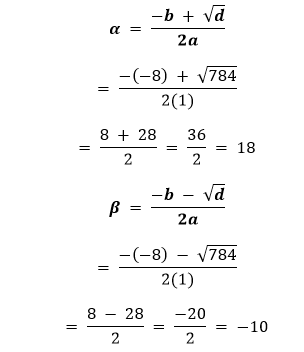
But, x cannot be negative as that will result in the square of smaller number to be negative which is not possible. Therefore, x = 18.

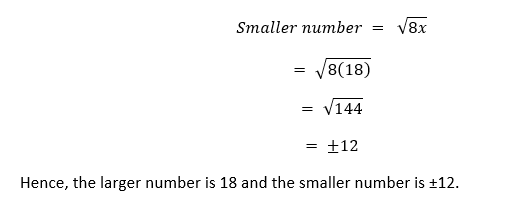
8. A train travels 360 km at a uniform speed. If the speed had been 5 km/h more, it would have taken 1 hour less for the same journey. Find the speed of the train.

Solution

Let the speed of the train (in km/h) = x.

Distance travelled = 360 km

We know that,

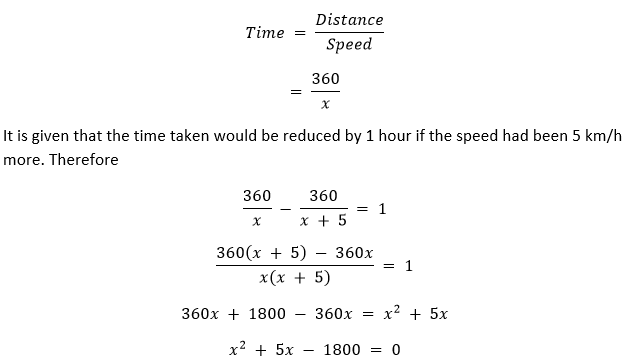
On comparing the given quadratic equation with ax2 + bx + c = 0, we get

a = 1, b = 5 and c = -1800

Find the discriminant

d = b² - 4ac
d = (5)² - 4(1)(-1800)
d = 25 + 7200
d = 7225

Since, d > 0.

Therefore, two real roots α and β exist for the given equation.

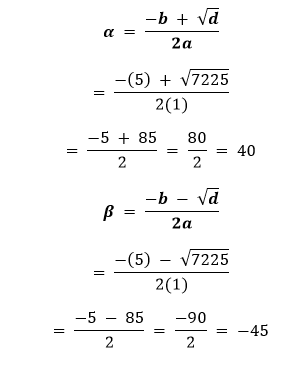
9. wo water taps together can fill a tank in 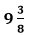hours. The tap of larger diameter takes 10 hours less than the smaller one to fill the tank separately. Find the time in which each tap can separately fill the tank.

Solution

Let the time taken by the tap of larger diameter to fill the tank (in hours) = x

Then the time taken by the tap of smaller diameter to fill the tank (in hours) = x + 10

Amount of water filled in the tank by the tap of smaller diameter in an hour = 1/(x + 10)

Amount of water filled in the tank by the tap of larger diameter in an hour = 1/x

It is given that if the two taps can fill the tank in 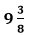hours = 75/8 together. Therefore

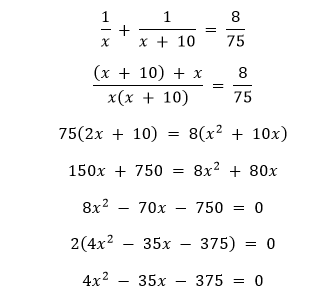
On comparing the given quadratic equation with ax² + bx + c = 0, we get

a = 4, b = -35 and c = -375

Find the discriminant

d = b² - 4ac
d = (-35)² - 4(4)(-375)
d = 1225 + 6000
d = 7225

Since, d > 0.

Therefore, two real roots α and β exist for the given equation.

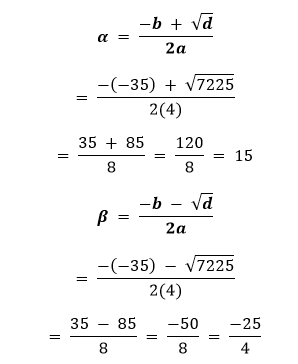
But time taken cannot be negative, so x = -25/4 is rejected.

Therefore x = 15

Hence, time taken by tap of larger diameter = 15 hrs

and time taken by tap of smaller diameter = 15 + 10 = 25 hrs.

10. An express train takes 1 hour less than a passenger train to travel 132 km between Mysore and Bangalore (without taking into consideration the time they stop at intermediate stations). If the average speed of the express train is 11km/h more than that of the passenger train, find the average speed of the two trains.

Solution

Let the average speed of the passenger train (in km/h) = x.

Then the average speed of the express train (in km/h) = x + 11

Time taken by passenger train to cover 132 km = 132/x

Time taken by express train to cover 132 km = 132/(x + 11)

It is given that express train takes 1 hour less to complete the journey. Therefore

On comparing the given quadratic equation with ax2 + bx + c = 0, we get

a = 1, b = 11 and c = -1452

Find the discriminant

d = b² - 4ac
d = (11)² - 4(1)(-1452)
d = 121 + 5808
d = 5929

Since, d > 0.

Therefore, two real roots α and β exist for the given equation.

But the speed of the trains cannot be negative, so x = -44 is rejected.

Hence, average speed of passenger train = 33 km/h

and average speed of express train = 33 + 11 = 44 km/h

11. Sum of the areas of two squares is 468 m². If the difference of their perimeters is 24 m, find the sides of the two squares.

Solution

Let the length of side of larger square (in m) = x

Perimeter of smaller square = 4x - 24

Then the length of side of smaller square = (4x - 24)/4 = x - 6

The sum of the area of both of the squares is given to be 468 m², therefore

x² + (x - 6)² = 468
x² + 36 + x² - 12x = 468
2x² - 12x - 432 = 0
2(x² - 6x - 216) = 0
x² - 6x - 216 = 0

On comparing the given quadratic equation with ax2 + bx + c = 0, we get

a = 1, b = -6 and c = -198

Find the discriminant
d = b² - 4ac
d = (-6)² - 4(1)(-216)
d = 36 + 864
d = 900

Since, d > 0.

Therefore, two real roots α and β exist for the given equation.

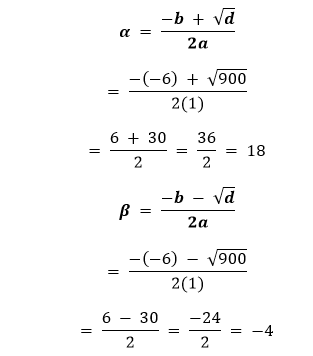
But, length cannot be negative, so x = -4 is rejected.

Hence, side of the larger square = 18 m,

and side of the smaller square = x - 6 = 18 - 6 = 12 m.

Exercise 4.4

1. Find the nature of the roots of the following quadratic equations. If the real roots exist, find them:

1. 2x² - 3x + 5 = 0
2. 3x² - 4 √ 3 x + 4 = 0
3. 2x² - 6x + 3 = 0

Solution

I. On comparing the given quadratic equation with ax² + bx + c = 0, we get

a = 2, b = -3 and c = 5

Find the discriminant

d =? b?² - 4ac
d = (-3)² - 4(2)(5)
d = 9 - 40
d = -31

Since, d < 0.

The roots for the given equation will be imaginary.

II. On comparing the given quadratic equation with ax2 + bx + c = 0, we get

a = 3, b = -4√3 and c = 4

Find the discriminant

d = b² - 4ac
d = (-4√3)² - 4(3)(4)
d = 48 - 48
d = 0

Since, d = 0.

The roots for the given equation will be real and equal.

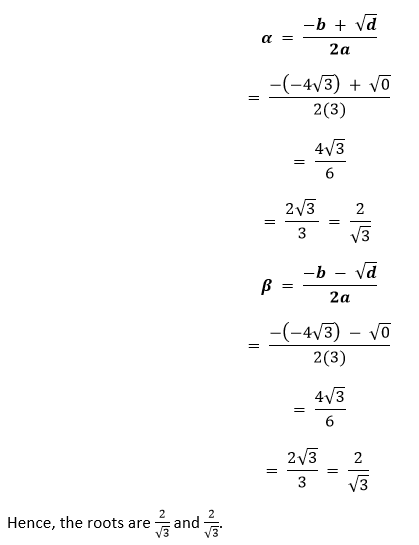
III. On comparing the given quadratic equation with ax² + bx + c = 0, we get

a = 2, b = -6 and c = 3

Find the discriminant

Since, d > 0.

The roots for the given equation will be real.

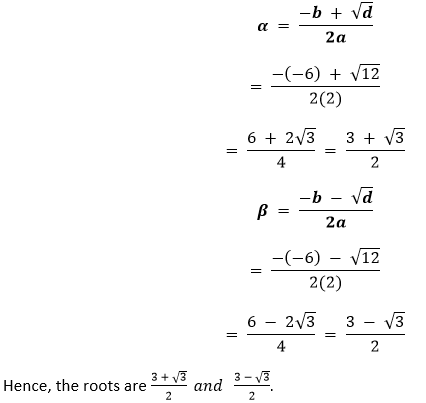
2. Find the values of k for each of the following quadratic equations, so that they have two equal roots.

1. 2x² + kx + 3 = 0
2. kx (x - 2) + 6 = 0

Solution

I. Since, it is given that the given equation has equal roots. Therefore, discriminant will be 0.

On comparing the given quadratic equation with ax² + bx + c = 0, we get

a = 2, b = k and c = 3

II. kx(x - 2) + 6 = 0

kx² - 2kx + 6 = 0

Since, it is given that the given equation has equal roots. Therefore, discriminant will be 0.

On comparing the given quadratic equation with ax² + bx + c = 0, we get

a = k, b = -2k and c = 6

d = b² - 4ac
0 = (-2k)² - 4(k)(6)
0 = 4k² - 24k
24k = 4k²
24 = 4k
k= 6

3. Is it possible to design a rectangular mango grove whose length is twice its breadth, and the area is 800 m²? If so, find its length and breadth.

Solution

Let the required rectangular mango grove have length of x m.

Then its breadth = x/2 m.

Area of rectangle = length × breadth

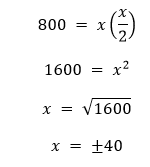
But length cannot be negative, so x = -40 is rejected.

Hence, the length of rectangular mango grove = 40 m,

and breadth of rectangular mango grove = 40/2 = 20 m.

4. Is the following situation possible? If so, determine their present ages.

The sum of the ages of two friends is 20 years. Four years ago, the product of their ages in years was 48.

Solution

Let the present age of one friend (in years) = x.

Then the present age of the other friend = 20 - x.

The ages of the two friends four years ago would be (x - 4) and (20 - x - 4) respectively.

The product of their ages 4 years ago is given to be 48. Therefore

(x - 4)(16 - x) = 48
16x - x² - 64 + 4x = 48
-x² + 20x - 64 - 48 = 0
x² - 20x + 112 = 0

On comparing the given quadratic equation with ax2 + bx + c = 0, we get

a = 1, b = -20 and c = 112

Find the discriminant

d = b² - 4ac
d = (-20)² - 4(1)(112)
d = 400 - 448
d = -48

Since, d < 0.

The roots for the given equation do not exist.

Hence, the given situation is not possible.

5. Is it possible to design a rectangular park of perimeter 80 m and area 400 m²? If so, find its length and breadth.

Solution

Let the length of the rectangular park = x m.

Perimeter of rectangular park = 80

80 = 2(length) + 2(breadth)

80 - 2x = 2(breadth)

Then, breadth of rectangular field = (80 - 2x)/2 = 40 - x

Area = Length × Breadth

400 = x(40 - x)
400 = 40x - x²
x² - 40x + 400 = 0

On comparing the given quadratic equation with ax2 + bx + c = 0, we get

a = 1, b = -40 and c = 400

Find the discriminant

d = b² - 4ac
d = (-40)² - 4(1)(400)
d = 1600 - 1600
d = 0

Since, d = 0.

Therefore, roots are real and equal for the given equation.

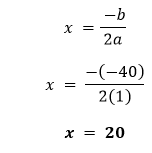
Hence, length of the rectangular park = 20 m.

Breadth of the rectangular park = 40 - x = 40 - 20 = 20 m.