Derivation of Equations of MotionIntroduction:Before discussing the equations of motion, we need to understand what is meant by the term 'motion'. Motion is the phenomenon of a change in an object's position with respect to time. In simpler terms, it refers to the movement or displacement of an object from one place to another. Motion can occur in various forms, such as linear, circular, periodic, or random, depending on the path the object takes and the forces acting upon it. We witness and partake in motion every day and every minute of our life. The movement of your hand or arms to pick up something, the object that is picked up and placed somewhere else, walking from one place to another, and driving in a car or any other vehicle, are all examples of some kind of motion we witness every day. Motion is prevalent in the universe, from the motion of celestial bodies like planets and stars to the motion of atoms and particles at the subatomic level. Observing and comprehending motion is essential for many scientific fields, engineering, and everyday life applications. It is a crucial concept for understanding the dynamics of the world around us and has played a central role in the development of various scientific theories and technological advancements. The study of motion enables us to understand how objects behave under the influence of forces, allowing us to predict their future positions and velocities based on initial conditions and applied forces 
Newton's Law of Motion:Newton's laws of motion are three fundamental principles that laid the foundation for classical mechanics and revolutionized our understanding of how objects move. These laws were formulated by Sir Isaac Newton in his work published in 1687. As the equations of motion are based on Newton's law, it is important first to grasp what the said laws dictate. Newton's 1st Law of Motion:The first law, also known as the law of inertia, states that: An object at rest will remain at rest, and an object in motion will continue to move with a constant velocity in a straight line unless acted upon by an external force. In simpler terms, an object will maintain its state of motion (whether at rest or moving with a constant speed in a straight line) unless an external force acts on it. This law implies that the natural tendency of an object is to resist changes in its motion, which is often referred to as inertia. 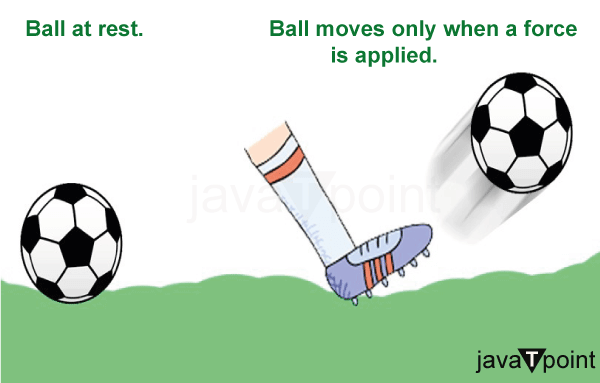
Newton's 2nd Law of Motion:The second law states that: The acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass. Mathematically, Newton's second law is expressed as F = m*a, where:
This law tells us that the force acting on an object is responsible for changing its state of motion. Larger forces cause greater acceleration, while more massive objects require larger forces to produce the same acceleration. Newton's 3rd Law of Motion:The third law of motion states that: For every action, there is an equal and opposite reaction. This law states that when two objects interact, the force exerted by one object on the other is matched by an equal but opposite force exerted by the second object on the first. In other words, if object A exerts a force on object B, object B simultaneously exerts an equal and opposite force on object A. Newton's laws of motion have had a profound impact on the development of physics and have become the cornerstone of classical mechanics. They are fundamental to understanding and predicting the motion of objects, from simple everyday situations to complex interactions in celestial mechanics and engineering applications. These laws continue to be taught and applied in various scientific fields and remain crucial for our understanding of the physical world. First Equation of Motion:The equations of motion are crucial principles in classical mechanics that describe the behavior of a particle in response to external forces. In one dimension, these equations establish a relationship between the particle's position, velocity, and acceleration as functions of time. The first equation states that the velocity of the particle is the time derivative of its position, representing how quickly the particle's position changes with time. Derivation:Let us consider an object with an initial velocity 'u'. After time 't' it accelerates to a final velocity of 'v'. The acceleration is 'a'. According to the definition of acceleration: acceleration (a) = Change in velocity/time taken (t) Now, change in velocity = final velocity (v) - initial velocity (u) This means: a = (v - u) / t Cross multiplying t with a, we get: a*t = v - u After rearranging the above equation, we get: v = u + a*t This is the first equation of motion. Second Equation of Motion:The second equation of motion describes the position of a particle as a function of time when the particle is subjected to constant acceleration. The equation is also known as the "position-time" equation and is one of the crucial equations of classical mechanics. The equation describes how the position of the particle changes with time when it undergoes constant acceleration. The equation assumes that the acceleration experienced by the particle remains constant throughout the motion. This means that the acceleration does not change with time and is the same at any given instant. Mathematically it is represented as: x = u * t + (1/2) * a * t 2 Derivation:Let us consider an object with an initial velocity 'u'. After time 't' it accelerates to a final velocity of 'v' and travels 'x' amount of distance. The acceleration is 'a'. The average velocity for this object would be: (u + v)/2 Now, we know that the distance traveled is the average velocity multiplied by the time taken: x = [(u + v)/2]*t From the first equation of motion, we know that: v = u + a*t Using this, our equation becomes: x = [(u + u + a*t)/2]*t x= (2u*t + a*t2)/2 x = u*t + ½(a*t2) This is the second equation of motion and is a crucial tool in classical mechanics and plays a significant role in understanding the motion of particles subjected to constant acceleration. It is widely used in various physics and engineering applications, such as analyzing the motion of projectiles, studying the motion of vehicles, and describing the dynamics of celestial bodies. Third Equation of Motion:The third equation of motion shows how the final velocity of the particle (v) is related to its initial velocity (u) when subjected to constant acceleration. The final velocity is obtained by squaring the initial velocity, adding twice the product of the acceleration and the displacement, and then taking the square root. It is also known as the "velocity-time" equation. Similar to the first and second equations of motion, the third equation assumes that the acceleration experienced by the particle remains constant throughout the motion. Mathematically, it is represented as: v2 - u2 = 2a*x Derivation:Using the first equation: v = u + a*t v - u = a*t t = (v-u)/a Now, substitute this value of t in the second equation of motion: x = u*t + ½(a*t2) x = u*(v-u)/a +½ [a*(v-u)2]/a2 x = (v*u - u2)/a + (v2 - 2*v*u + u2)/2a x = (2*u*v - 2*u2 + v2 - 2*u*v + u2)/2a 2ax= v2 - u2 or v2 - u2 = 2ax This is the third equation of motion. The third equation of motion complements the first two equations by providing a way to calculate the final velocity of an object under constant acceleration given its initial conditions (velocity and displacement). This equation is valuable for understanding the dynamics of moving objects and is used in various physics and engineering applications, such as calculating the final speed of a vehicle after a certain distance traveled or predicting the velocities of projectiles in projectile motion problems. Conclusion:The equations of motion are derived from Newton's second law of motion and velocity can be calculated by integrating the acceleration and position can be calculated by integrating the velocity. These equations provide a mathematical description of the motion of a particle under the influence of external forces. These equations, along with Newton's second law relating force and acceleration, provide a powerful framework for understanding and predicting the motion of objects in various scenarios, from simple linear motion to more complex dynamics involving multiple dimensions and interactions.
Next TopicDoppler Effect
|
 For Videos Join Our Youtube Channel: Join Now
For Videos Join Our Youtube Channel: Join Now
Feedback
- Send your Feedback to [email protected]
Help Others, Please Share









