1 Radian to Degrees

There are two alternative systems available to measure an angle. A radian, used to measure angles, is the angle formed at the centre of a circle by an arc with a length equal to the circle's radius. A degree is a different unit used to measure angles. 1 radian equals 57.296 degrees when expressed in terms of degrees. There is a formula that may be used to change the unit of measurement from radians to degrees.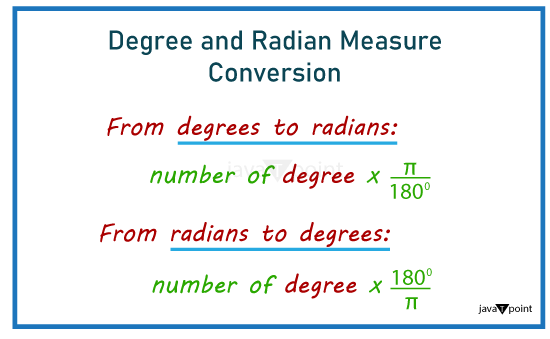

Definition of Radian

An SI unit that is useful in measuring angles is the radian. Additionally, it is the accepted unit of measurement for angular distance in all areas of mathematics. The measurement in radians of the angle an arc occupies is numerically equal to the length of the arc in the unit circle.

The unit, however, was formerly a SI unit by the supplement. Since 1995, the radian has been an SI-derived unit. Separately, the solid angle measurement uses the steradian as the SI unit. Additionally, the sign rad is most frequently used to symbolize the radian. C serves as its alternate symbol. Either a superscript R, a superscript C, or a superscript R.

In mathematics, the radian measures the plane angle equal to 180/degrees, or around 57.2958 degrees. The abbreviation "rad" or, less frequently, the superscript "c" (for "circular measure") is used to indicate it. An angle of 1.2 radians, for example, would be expressed as "1.2 rad" or "1.2c" (the second symbol can be confused with a degree: "1.2°").

Formula of Radian

Firstly, One degree equals PI/180 radians, and one radian equals 180/PI degrees. Decide how many degrees you want to convert to radians by multiplying the number of degrees by PI/180 (for instance, 90 degrees = 90 x PI/180 radians = PI/2). Multiply the number of radians by 180/PI to convert the given number of radians to degrees.

Definition of Degree

The unit for measuring a plane angle is a degree, sometimes called an arc degree or a degree of arc. The symbol (°) is used to denote the degree. The angle that represents a full rotation is 360°. Although degrees are a commonly used unit of measurement, they are not a SI unit for angles.

1 Radian to Degrees Formula

One rotation is accomplished by moving the radius around the circle. After one full rotation, the angle that the radius occupies at the circle's centre is 2 radians. The conversion of 1 radian to degrees will be illustrated with the fact that 2 radians equal 360 degrees.

2π radians = 360°

Divide the above equation by 2,

⇒ π radians = 180°

Divide the above equation by π,

⇒ 1 radian = 180°/π

⇒ 1 radian = 57.296°

Hence, 1 radian to degrees measures 57.296°

How to Convert 1 Radian to Degrees?

Angles are measured in two different measurement systems: degrees and radians. The ratio of 1 radian to a degree allows us to convert any angle's measure from radians to degrees.

The conversion of radians to degrees can be done using the formula 'Angle in Radians × 180°/π = Angle in Degrees'. We know that 360° equals 2π radians, and this result can be used to convert 1 radian to degrees. 360° = 2π radians ⇒ 180° = π radians ⇒ 1 radian = 180°/π ⇒ 1 radian = 57.296°.