Squares 1 to 100

Squares play an important role in various fields of study, especially mathematics. To quickly solve mathematical issues, you must be able to recall squares. The Squares from 1 to 100 will be covered in this article along with the concept to determine them.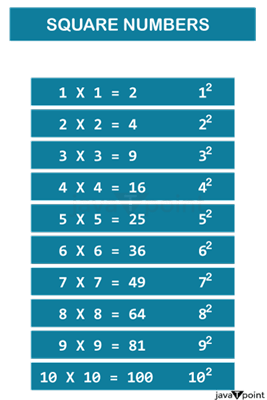

What is a Square?

The result of multiplying a number with that same number is known as the square of the original number. For example, to calculate the square of X, we need to multiply X by X itself, i.e., X x (multiplication sign) X or X^2.

Example:

• A square of 2 is 2 × 2 = 4
• A square of 3 is 3 × 3 = 9
• A square of 4 is 4 × 4 = 16
• A square of 5 is 5 × 5 = 25
• A square of 6 is 6 × 6 = 36, and so on.

Squares from 1 to 100

The square of 1 to 100 can be written in exponential notation as (X)^2, where x represents values starting from 1 and going up to 100

• Form of Exponent: (x)^2
• Maximum Value: 100^2 equals 10,000.
• Minimum Value: 1^2 = 1.

Squares from 1 to 100:

Let's read the squares below, which range from 1 to 100:

What characteristics do square numbers have?

The following characteristics of square numbers can be generalized based on what we saw in the previous section on the list of squares from 1 to 100:

• Square numbers (results) typically have a unit place ending with 0 (especially an even number of zeros or 00), 1, 4, 5, 6, or 9.
• A number's square result value will always have a 1 at the end in case the original number ends with 1 or 9.
• A number's square result value will always have a 6 at the end in case the original number ends with 4 or 6.
• A number ending with a double zero (or with an even number of zeros/ 00) will be a perfect square.
• A number ending in 2, 3, 7 or 8 cannot be a perfect square.
• A perfect square's square root value is always a natural integer.

How to determine the values of Squares from 1 to 100?

We may use any of the following approaches to compute the squares from 1 to 100:

Approach 1: Simple Multiplication

By multiplying the number by itself, we may obtain the square of the original number, be it small or large. For example, using this technique, we can determine that square of 8 (i.e., 8x8) equals 64. In other words, the final value or resultant value "64" is the square of the value "8". For lesser (or small) numbers, this strategy works effectively.

Approach 2: Applying Fundamental Algebraic Identities

This method is often used to simplify large values and then find their squares. To find the square of 49, for instance, we can write 49 as follows:

• Choice A is (40 + 9)^2
• Choice B is (50 - 1)^2

The fundamental algebraic identity formula (a±b)^2 = (a²+b²±2ab) is used in the next step to obtain the following results:

• Choice A, [40² + 9²+ (2×40 × 9)], and
• Choice B, [50² + 1²- (2×50 × 1)].

It is clear that 'a' represents the value '40' and 'b' represents the value '9' for choice A, whereas in choice B, 'a' represents the value '50' and ' b' represents the value '1'. Therefore, we get the following results:

• Choice A: (1600 + 81 + 720) = 2401, and
• Choice B: (2500 + 1 - 100) = 2401

We can see that the value of 2401 is the result of both the choices (equations) used to find the value of square 49.

Example: Use of Squares in Real Life

Question: There is a round tabletop with a radius of 50 inches. What will be the area of that tabletop? [Use π = 3.14]

Solution:
As we know that the area of a circle is πr^2, where r is the radius. Therefore, Area of tabletop = π(50)2

Using the square values from 1 to 100 chart, 502 = 2500.
So, the Area of the tabletop = 2500π
Since the value of π is 3.14, the Area of the tabletop = 2500 × 3.14 = 7850
Hence, the tabletop's area is 7850 inches2.

The Bottom Line

As an outcome, investigating the idea of squares from 1 to 100 shows remarkable patterns and insights into the field of mathematics. The total number of perfect square numbers in this range, 10, has led us to the conclusion that perfect squares are widely distributed within numbers. These squares reveal an unusual arrangement, whether represented in a square grid or when visualized on a number line, in addition to having distinctive characteristics, such as being the result of a number multiplied by itself. In addition, a study of squares reveals relationships with a number of mathematical ideas, including factors, divisibility, and even geometry. Overall, the study of squares from 1 to 100 is an excellent illustration of the variety and beauty of mathematics and how it has significantly influenced how we see the world.