How to Divide FractionsIn arithmetic, dividing fractions is much tricky but not difficult. If we ignore the reciprocal it is similar to the multiplication of fractions. In this section, we will learn how to divide fractions. We can also divide a fraction by applying the division symbol (÷) between dividend and divisor. The fraction that is left of the division symbol is called the dividend, and the fraction that is right to the division symbol is called the divisor. 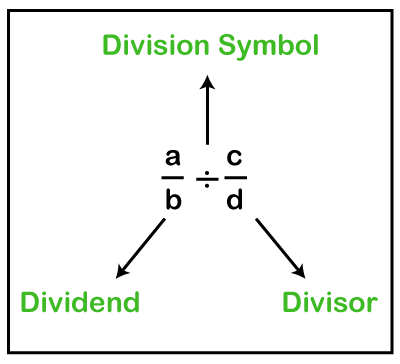
Methods of Dividing FractionsThere are two methods to divide fractions.
We can use both the methods in the following cases:
Invert and Multiply MethodIt is the easiest and commonly used method to divide fractions. In this method, first, we find the reciprocal (invert) of the divisor. After that multiply the dividend by the reciprocal. Do not use this for a complex problem.
Suppose, 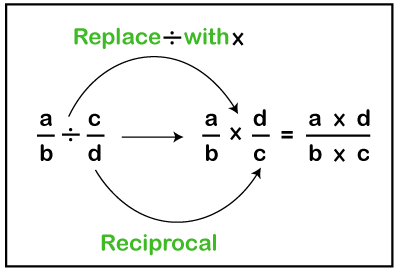
Suppose, 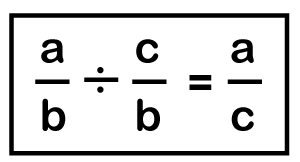
It is because if we find the reciprocal of the divisor, we get 
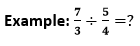
Solution: In the above question, Step 1: Find the reciprocal of the divisor. 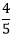
Step 2: Multiplying dividend by the reciprocal. 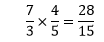
Hence, on dividing Common Denominator MethodIn this method, we make the denominators of both fractions the same. It is the most correct method to divide fractions. Find a common denominator and convert each fraction to its equivalent value.
Note: When converting the fraction to its equivalent value, multiply the same number in numerator and denominator.
Solution: In the above question, Step 1: To find the common denominator, we will multiply 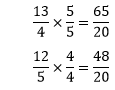
Step 2: Divide the numerator of the fractions. 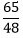
Hence, on dividing 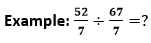
Solution: In this question, Hence, on dividing Dividing Fraction and Whole NumberWe can also divide a fraction by whole number or vice-versa. While dividing a fraction by the whole number, convert the whole number into a fraction by putting 1 in the denominator. It does not change the value. After that solve the question by using any one method that we have discussed above. 
Solution: In this question, the divisor is not in the fractional form. First, we will convert the whole number (7) into a fraction, i.e., 
Step 1: Find the reciprocal of the divisor. 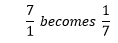
Step 2: Multiplying dividend by the reciprocal. 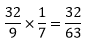
Hence, on dividing 
Solution: In this question, the dividend is not in the fractional form. First, we will convert the whole number (13) into a fraction, i.e., 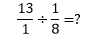
Step 1: Find the reciprocal of the divisor. 
Step 2: Multiplying dividend by the reciprocal. 
Hence, on dividing 13 by Dividing Mixed FractionWhen dealing with the mixed fraction, follow the steps given below:

Solution: Step 1: Convert the mixed fraction into an improper fraction, that is: 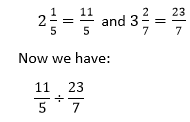
Step 2: Find the reciprocal of the divisor, that is: 
Step 3: On multiplying the dividend fraction by reciprocal, we get: 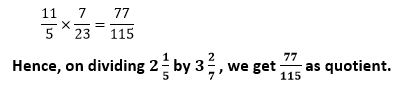
Dividing More Than Two FractionsWe use the inverse and multiply method when any number of fractions is given for division. After this simplify the fraction, if required. 
Solution: Write the first fraction the same, and apply inverse, and multiply method in other fractions. Simplify the fraction, if required. 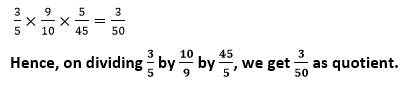
Next TopicHow to Multiply Fractions
|
 For Videos Join Our Youtube Channel: Join Now
For Videos Join Our Youtube Channel: Join Now
Feedback
- Send your Feedback to [email protected]
Help Others, Please Share





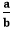 and
and 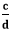 are two fractions and we want to divide
are two fractions and we want to divide 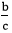 . Now multiply the dividend by the reciprocal, we get:
. Now multiply the dividend by the reciprocal, we get: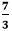 is the dividend and
is the dividend and 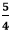 is the divisor.
is the divisor.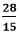 as quotient.
as quotient.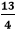 is the dividend and
is the dividend and 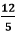 is divisor.
is divisor.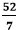 is the dividend and
is the dividend and 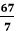 is the divisor having the same denominator. So, we will divide the numerator only, i.e.
is the divisor having the same denominator. So, we will divide the numerator only, i.e. 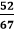 .
. . Now we have converted the question into the following:
. Now we have converted the question into the following: by 7, we get
by 7, we get 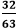 as quotient.
as quotient.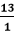 . Now we have converted the question into the following:
. Now we have converted the question into the following: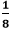 , we get 104 as quotient.
, we get 104 as quotient.




