Cubes 1 to 20

A cube is a result of a number being multiplied by itself, itself, and then itself again (typically multiplied three times, i.e., n x n x n). The geometric representation of a number as a cube in three dimensions offers a unique insight into its magnitude.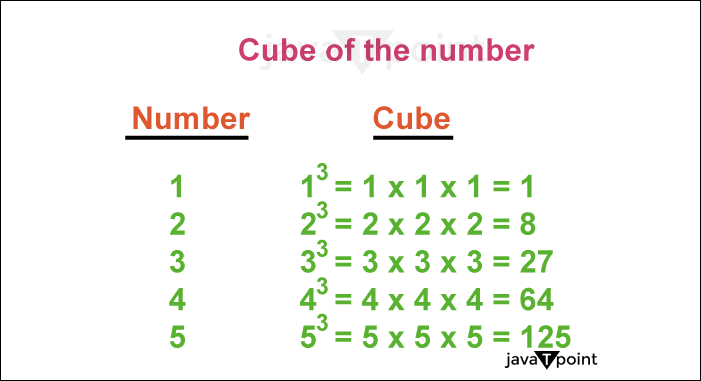

The idea of cubes of numbers from 1 to 20 will be explored in detail in this article. We will find an exciting pattern that reveals features and linkages by computing the cubes of these integers. These cubes have numerous practical uses and significance in mathematics and science.

As the cube of a number represents the volume of a cube with sides of that length, studying cubes of numbers offers insights into the concept of volume. The cubes of numbers are also used in algebra, calculus, and number theory as a foundation for resolving equations, identifying patterns in series, and investigating integer properties.

In addition to its uses in mathematics, cubes are helpful in physics, engineering, and computer science. The cubic function is a crucial element of mathematical simulations and models, offering an understanding of physical events and supporting the creation of algorithms and structures.

Definition

The cubes of numbers refer to the result of multiplying a number by itself twice. It is the mathematical operation of raising a number to the power of 3.

In mathematical terms, if we have a number 'n', the cube of 'n' is obtained by multiplying 'n' by itself twice. It can be represented as 'n³' or 'n × n × n'.

How to calculate cubes?

Follow the instructions below to find the cube of any number:

Step 1: Specify as 'n' the number you want to find the cube of.

Step 2: Multiply the number twice by itself (n x n x n).

Step 3: The result came is the cube of that number.

Example: Calculate the cube of the number 7.

Step 1: Let n = 7

Step 2: n³ = 7×7×7

Step 3: n³= 343

So, the cube of the number 7 given here is 343.

Why are they called cube numbers?

They are called cube numbers (or cubed numbers) because they can be used to determine the volume of a cube. A cube is a three-dimensional object with sides that are all the same size, thus you can determine its volume by multiplying the side length by itself twice (or "cubing" it).

It is simple to "cube" (multiply by itself twice) one of a cube's sides to calculate its volume because its length, height, and width are all the same.

For instance, a cube with sides measuring 2 cm would have an 8 cm³ volume (because 2³ = 8). Reversed, we would know that a cube's sides would each be 3 cm long if we knew its volume was 27 cm³ (because 3³ = 27).

Advantages of Cubes

The number cubes have significant benefits in a variety of mathematical applications.

• Cubing a number helps compute three-dimensional values and represent the volume of a cube with that side length in geometry.
• Recognizing and making use of cubes makes it easier to solve and factor polynomial expressions, which in turn simplifies challenging algebraic equations.
• Number Sequences, Prime Numbers, and Integer Properties can all be identified by examining the patterns and correlations that emerge from the analysis of number cubes.
• Understanding the exponential development of number cubes makes it easier to understand its patterns, which is helpful in areas like finance and scientific modelling.

Table of Cubes of Numbers from 1 to 20

Learning the cubes from 1 to 20 can assist students in recognizing all perfect cubic values up to four digits and approximating a cube root by interpolating between previously learned cubes. The table below lists the values for cubes 1 to 20.

The mathematical and non-mathematical applications of number cubes are invaluable. They aid in the visualization of volumes, the solution of algebraic problems, the discovery of numerical patterns, the comprehension of exponential growth, and the improvement of programming computations. Accepting the idea of cubes creates a more profound comprehension of mathematical ideas and improves problem-solving skills.