What is 10 to the 6th Power?

10 to the sixth Power, written as 10^6, represents the mathematical procedure known as exponentiation. It entails increasing the base number 10 to the Power of 6, equivalent to multiplying ten by itself six times. In this article, we will look at the notion of exponentiation, the relevance of 10^6, and numerous real-world situations where such big numbers are important.

What is an Exponentiation?

Exponentiation is a fundamental mathematical operation that allows us to represent repeated multiplication efficiently and concisely. The exponent, also known as the Power, reflects the number of times the base has been multiplied.

Notation of 10 to the Power 6

There are several ways to describe this, but the ones you'll encounter most frequently are as follows:

1. The exponent will either be represented by a superscript (which makes it smaller and somewhat above the base number) or
2. By the caret symbol (^). The caret might be helpful when using superscript is not be desirable or necessary.

Calculation of 10 to the Power 6

The base is 10 in this question (10^6), and the exponent is 6. As a result, 10^6 can be computed as follows:

10^6 = 10 × 10 × 10 × 10 × 10 × 10 = 1,000,000

Hence, 10 to the 6th Power equals 1,000,000.

To gain a better understanding of the magnitude of 10^6, let's explore some comparisons and contexts. First and foremost, it is vital to remember that 10^6 equals one million. In the International System of Units (SI), the prefix "mega-" is used to represent the value of one million.

10^6 falls within the realm of substantial numerical values. It is significant when dealing with large-scale phenomena or quantities involving many units.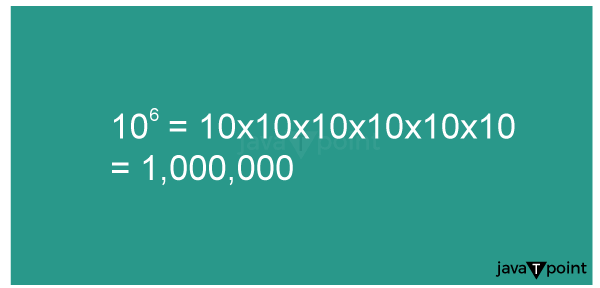

Uses of 10 to the Power 6

Let's look at a few examples of how 10^6 is used to indicate meaningful quantities:

Population: The population of cities, regions, or nations is frequently measured in millions. Major cities, such as New York City or Tokyo, have populations in the millions. Similarly, nations with populations in the millions include Singapore and Luxembourg.

Data Storage: Data storage capacity is measured in bytes in computers. According to rate of conversion recommended by the International Electrotechnical Commission (IEC), one megabyte (MB) equals to 1 million (10^6) bytes. Similarly, 1 Gigabyte is equal to 1000 MBs (or 10^6 bytes). Such large amount of storage can carry large amounts of data, such as lengthy text, multiple high-resolution photos, or a short movie.

Time: In certain settings, the number 10^6 denotes periods. For example, one million seconds is approximately equivalent to 11.6 days. This number is frequently used to measure the duration of periods or occurrences.

Money: Financial statistics often involve values in the millions. For example, a person's net worth, business earnings, or the cost of large-scale projects might be worth millions.

Scientific Notation: Scientists and mathematicians frequently use scientific notation to express very large or extremely small numbers..

Negative Exponents

It's important to remember that the concept of exponentiation is applicable to both smaller and larger integers. Exponents can be either positive or negative. In case of negative exponent, the reciprocal of the base is raised to the absolute value of the exponent.

For example, if we assume 10^-6, the computation is as follows:

10^-6 = 1 / (10 × 10 × 10 × 10 × 10 × 10) = 0.000001

In this case, 10^-6 equals 0.000001 or one divided by one million.

Exponents encompass more than just integers. They can also be fractional or decimal numbers, which introduces the idea of roots and powers. For example, the square root () corresponds to an exponent of 1/2, the cube root (3) to an exponent of 1/3, and so on.

Conclusion

In conclusion, the mathematical idea of 10^6 is a basic illustration of exponentiation. It symbolizes the value produced when you multiply ten by itself six times, giving you a value of one million. Understanding huge numbers and their exponential representation is essential for understanding many facets of our world, from economics and scientific notation to demographics and data storage.