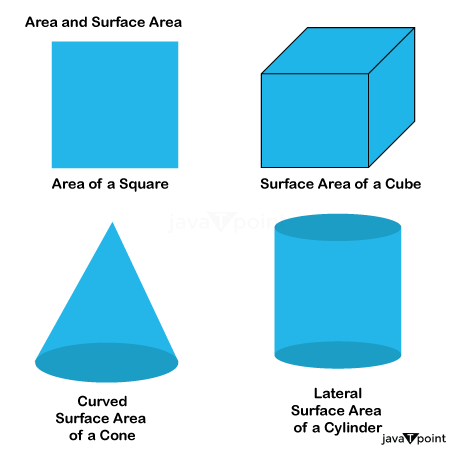
Difference Between Area and Surface Area

The field of Geometry, which is a branch of mathematics, deals with the measurements (area, perimeter, volume, surface area, etc.) of different types of shapes (like Square, Pyramid, Rectangle, Cone, etc.), whether they belong to two-dimension (2-D) or three-dimension (3-D). There are two concepts, "Area" and "Surface Area," in geometry that usually confuse the students and those unfamiliar with these concepts. Well, they may seem similar, but they have different meanings and applications, so in this article, we will learn about the concepts of area and surface area, their definitions, differences, properties, and applications, and end this article with a conclusion.

Definitions of Area

The area is a fundamental concept in geometry that refers to the amount of space enclosed by a two-dimensional (2-D) shape; we can also say that area is a measure of the extent of a flat surface within a boundary. Whenever we discuss area, we typically deal with (2-D) shapes like squares, rectangles, triangles, circles, and irregular polygons. Every shape has its own different area; for instance, the area of a square differs from that of a rectangle. As the area is related to 2-D shapes, these shapes have definite lengths and widths; the area of any shape can be calculated with the product of the length and width of that shape.

Units

The unit of measurement for an area depends on the context (particularly, depends on the country in which we are in) but is typically square units such as square meters (m²), square feet (ft²), or square centimeters (cm²).

Area of Some Definite Shapes

Applications of Area

The concept of area, which is the measure of the size of a two-dimensional region or shape, has a wide range of applications in various fields, and some of them are discussed below:

• Mathematics: The concept of area is the base of geometry and trigonometry, where it is used to solve problems that involve shapes such as triangles, circles, rectangles, and polygons; calculus also uses the concept of area to find the area under a curve.
• Architecture: Architects use the concept of area to design and plan the layout of buildings and calculate the materials needed for construction.
• Physics: The area is used to calculate quantities such as the area of a beam's cross-section, surface area, and capacitor plate's area.
• Engineering: Engineers use the concept of area to design structures such as buildings, bridges, and roads. They also use it to calculate the cross-sectional area of pipes, cables, and other components.
• Agriculture: In agriculture, the area concept determines the land needed for crops and calculates the yield per unit area.
• Cartography: Maps represent a region, and the area of a region is an important factor in mapmaking; cartographers use the concept of area to accurately describe (map) the size of different regions on a map in a particular proportion.

Definitions of Surface Area

The surface area of an object refers to the area of the surface of a three-dimensional (3-D) object or shape. When we deal with three-dimensional objects with two-dimensional faces or simply surfaces, the surface area is calculated by adding up all the areas of all the individual faces from which that shape or figure is made. There are so many examples of shapes that are 3-D, such as cubes, cuboids, cones, and cylinders. To ascertain the surface area of these 3-D objects, one must add up the areas of their constituent faces, employing well-known basic area formulas applicable to these familiar 2-D shapes.

For better understanding, we can consider an example of a cube that has six faces, so the surface area of the cube is derived from adding all the areas of all six faces; as we know, the face of a cube is a square, and this implies the surface area of a cube is equal to six times the area of a square (face of a cube).

Classification of Surface Area

On the basis of which part of a shape or object we see, we can divide the surface area of an object into three parts, which are discussed below:

1. Total Surface Area

The total surface area encompasses all faces, including the base of that object or shape. In the case of a right circular cylinder, adding the lateral surface area or curved surface area to the areas of both circular bases gives the total surface area of the cylinder.

2. Curved Surface Area

This variant of the area encompasses all the curved surfaces within an object (outer or inner surface). For instance, in the case of a right circular cylinder, the surface that connects the two bases of the cylinder is called the curved surface area of that cylinder.

3. Lateral Surface Area

Lateral surface area is equal to the sum of all the faces of an object or shape, with the exclusion of its top and bottom surfaces. In the context of a cube, the lateral surface area would be equivalent to the area of its four sides, i.e., 4 * area of the square (face of the cube).

It is important to note that the area is a measure of a 2-D object; for instance, when we talk about painting a side wall of a hall or room within a house, we are dealing with the 2-D measure of the surface area of the wall even though a house is a 3-D object, our main goal is to quantify the surface area of the wall. In this case, calculating the surface area of a hall allows us to determine the accurate quantity of paint required to cover all the walls effectively.

Units of Measurement

Surface area is also measured in square units, such as square meters (m²), square feet (ft²), or square centimeters (cm²).

Surface Area of Some Definite Shapes

Applications of Surface Area

• Painting a Box: Imagine you have a gift box and want to paint it. You have to calculate the amount of paint you need; you have to find the box's surface area, which includes all six sides. Knowing the surface area helps you estimate the amount of paint required.
• Wrapping Gifts: When wrapping a gift, you measure the object's surface area, such as a book or a toy. This concept helps you to cut the right amount of wrapping paper to cover the entire gift neatly.
• Heat Exchange: In cooling systems, like car radiators or refrigerator coils, surface area is important for efficient heat exchange. Larger surface areas allow for better cooling because more heat can be transferred to the surrounding environment.
• Geometry Education: In the geometry class, students learn about surface area by studying three-dimensional (3-D) shapes like cubes, prisms, and pyramids; they calculate surface areas to understand spatial relationships and solve geometric problems.
• Carpentry: Carpenters need to calculate the surface area of wooden panels, boards, or panels to determine how much paint or varnish is needed for a project, such as a wooden cabinet or a table.

Key Differences

Some Questions

Q: What does "area of a shape in a flat surface" refer to?

Answer: The area of the shape on a flat surface is the region or area covered by that shape, which determines its size.

Q: How would you define the "surface area of a solid"?

Answer: The surface area of a solid shape can be described as the measurement of all the exposed (which we can see) surfaces of that shape.

Q: What distinguishes "surface area" from "total surface area"?

Answer: Surface area particularly refers to the measurement of one face of a solid exposed (outside which we can see) within a three-dimensional plane; total surface area, on the other hand, is the sum of the areas of all the surfaces of the solid. For instance, in the case of a cube with six faces, its total surface area is shown by multiplying the surface area of one face by 6; since the faces of a cube are square, the formula for total surface area is 6 times the square of its side length (6 * side²).

Q: Is the concept of the "area of a rectangle" the same as that of a "cuboid"?

Answer: The area of a rectangle is determined by multiplying its length and Breadth, which is a two-dimensional measure; however, the area of a cuboid refers to its total surface area, which is equivalent to twice the sum of its faces (2 * [Length * Breadth + Breadth * Height + Length * Height]). Therefore, while both involve area calculations, they differ in shape and dimension.

Conclusion

We can say that area and surface area are basic mathematical concepts that play vital roles in various fields, from construction and engineering to science and design. In contrast, both concepts involve measuring space; their main difference comes in terms of dimensionality, types of shapes, and the formulas used for calculation.

The area focuses on two-dimensional shapes, measuring the extent of a flat surface, while surface area extends into three-dimensional space, encompassing the total area of all the surfaces enclosing a volume; understanding the distinction between these concepts is important for making accurate calculations and informed decisions in so many practical applications.